#femalecomposerfridays and enlivening interval work with Amy Beach's Dancing Leaves, Opus 102, No. 2
Each semester I try to develop new strategies for consistent representation of diverse voices in my theory classes. These strategies get worked into my regular course plans, and hopefully are positive steps towards a more inclusive music theory experience. But these are my own teaching goals—they get absorbed into the class, and the motivation behind them does not necessarily translate to students. Emphasizing and re-emphasizing the problems of representation in music theory is essential not only as a starting point for change, but for students to understand the context and need for this change. I decided to try something different this semester, something that would be a more visible method of showing both problems and positive change, but would also be structurally central and consistent. #femalecomposerfridays showcases the work of a female composer within a single class session per week. While this might seem like a huge time commitment in the scheme of class meetings, course structure, and curricular demands, it is essentially the same practice as having a dedicated “analysis day” each week. On #femalecomposerfriday I select a work that is aligned with that week’s course material and allows us to explore those concepts more closely in practice. Limiting these works to music by women is both a unique aspect of the course and a rewarding challenge for me. So far, students have responded positively and I’m hopeful they will maintain this enthusiasm throughout the semester.
I know some might think #femalecomposerfriday is somewhat excessive within the context of a theory class. But I think it’s just the start. Think big! Just four weeks in, we have already studied more music by women than the average theory class would cover in an entire semester.
I hope you will consider #femalecomposerfriday or a similar idea (#womencomposerwednesday?)in your classroom.
All this said, this post is mainly about Amy Beach, and a piece that’s great for in-class interval work. Many students in introductory-level theory courses require a good deal of practice in order to lay a solid foundation for future concepts, but interval spelling and identification can be especially tedious. Incorporating “real music” as much as possible in lieu of worksheets and endless sample problems can help make these rudiments a bit more engaging.
mm. 1 - 4
mm. 5 - 12
mm. 38 - 48
mm. 64 - 74
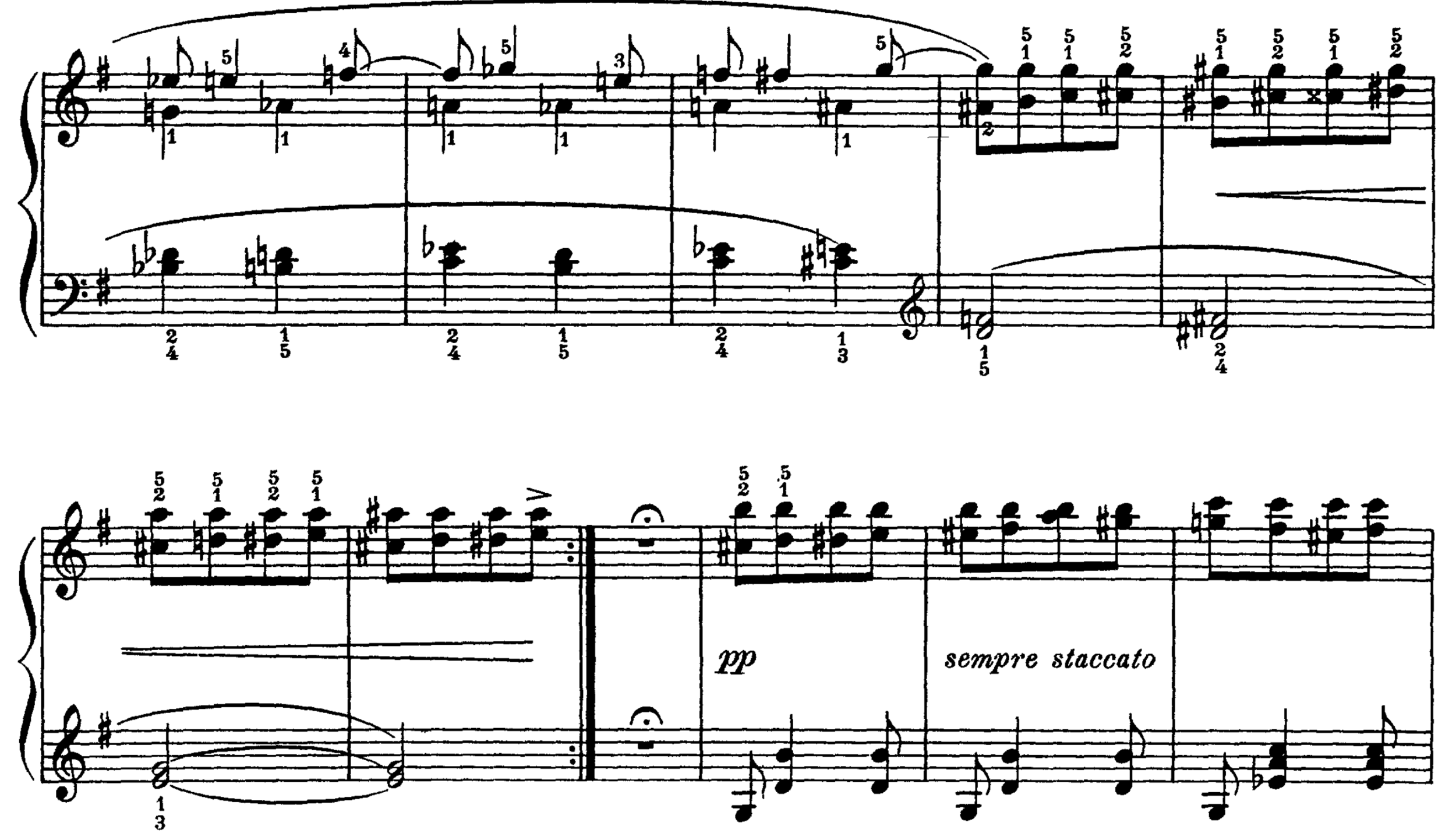
Amy Beach’s Dancing Leaves is my go-to piece for in-class interval identification work, for several reasons. The abundant chromaticism yields a wide range of intervallic spellings and presents opportunities to discuss enharmonic spellings as well as consonance and dissonance. Meanwhile, oblique motion throughout (especially through the use of pedal points with chromatically ascending or descending melodic lines) allows students to easily observe harmonic intervals increasing or decreasing by half step. Finally, the motivic use of intervals like the minor third helps illustrate the programmatic nature of the piece. Here are some of the aspects of the piece that I incorporate into our in-class interval work:
Spelling intervals (general): there is a wide variety of intervals in this short, accessible piece.
The chromatic four-measure introduction features mainly parallel motion descending minor thirds (m3) in the left hand. The second interval, an augmented second (+2) is a great point of discussion. Given we hear the parallel falling thirds (the falling leaves) as a unified gesture, it is useful to draw the distinction between the notation of the +2 and the aural effect of the interval. Students can be reminded that sometimes the way an interval appears (and should be identified) has different implications than those of sound.
In measures 5 - 10, oblique motion gives a fantastic visual of harmonic intervals increasing and decreasing by half step. For example, the first six intervals grow smaller in size (m7 - M6 - m6 - P5- dim5 - P4) while the following five grow larger (M2 - m3 - P4 - dim5 - dim6).
Similarly, in measures 41 - 44 oblique motion and quickly shifting pedal notes lead to a pattern of decreasing intervals: measure 41, dim7 - m6 - P5 - dim5; measure 42, m6 - P5 - dim 5 - P4; measure 43, m6 - P5 - dim5 - P4; measure 44, M6 - aug5 - P5 - aug4.
This pattern of decreasing intervals through oblique motion is also present in the last 9 measures of the piece, in which pedal notes arpeggiate the tonic triad as the chromatic melody line rises to a triumphant finish.
Looking at structural melodic intervallic relationships is also valuable. For example, in the left hand measures 1 - 4, both the upper and lower pitches descend a P5 by the end of the phrase. This P5 motion appears elsewhere as well, including an ascent in measures 5 - 6 (C# - G#); a descent in measures 11 - 12 (E - A and B - E); and repeated ascending P5’s in the continually rising chromatic alto line in the final measures.
Spelling intervals (specific): this piece is a great reference for harmonic augmented and diminished intervals. There is abundant use of tritones (expressed as +4 and dim5), as well as +2, +3, dim4, +5, dim7, and others. The substantial use of repeated, parallel-motion intervals (most frequently m3, but also P4 and P5) is also useful.
How do intervals relate to narrative? Most students find the imagery of this piece very accessible. Exploring how the whirling chromatic lines and parallel intervallic motion may work independently and together to suggest falling leaves is a rewarding way to conclude an interval-based discussion of this piece.